§ 2.3. Point symmetry.

If the manifold is a regular polyhedron, we can examine its point symmetries which preserve the center.

Figure 13. Square without/with point symmetry: The torus is mapped as a square to its cover, the Euclidean plane $R^{2}$ . Functional analysis employs a twofold Fourier series. Any reasonable function with domain the square A can be written as a twofold Fourier series. Periodicity restricts to the same functional values on parallel bounding edges of the square. If in addition we require the rotational 4fold symmetry of the square, the domain for a function is reduced to the sector shown in B.

For the interval $J$ we can ask for its point group symmetry operations preserving the center $x=0$ . The only non-trivial operation of this type is the reflection $R:x\rightarrow-x$ . With respect to functions on the interval $J$ we can ask for a basis of functions on $J$ invariant under this reflection. Clearly we must replace the exponential functions by the combinations $\cos(k_{n}x)=\frac{1}{2}(\exp(ik_{n}x+\exp(-ik_{n}x))$ , and the expansion eq.11 must be replaced by

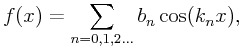			$\displaystyle f(x)=\sum _{{n=0,1,2...}}b_{n}\cos(k_{n}x),$		(12)
			$\displaystyle b_{n}=2\int _{{-1/2}}^{{1/2}}dx\: f(x)\cos(k_{n}x)$

These basis functions have both periodicity and reflection symmetry. The restriction to reflection symmetry reduces the set of basis of the Fourier series roughly by a factor two.

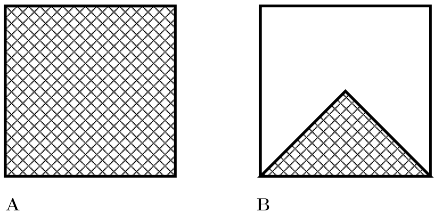
In Fig.13 we illustrate the effect of 4fold point symmetry of the square in the Euclidean plane, which is the universal covering of the torus. The domain of definition of functions is restricted from the full square A by 4fold point symmetry to the sector shown in B. It is easy to find the corresponding restriction on the basis functions eq. 13 for the functional analysis.